Extra Questions Answers for Class 6 Maths Chapter 2 Lines and Angles - Ganita Prakash
Chapter 2 Lines and Angles Extra Questions Answers for Class 6 Maths is provided here by studyrankers. All the questions are crafted by our experts by keeping in mind that all the important points must be covered. You can also Download PDF of Class 6 Maths Chapter 2 Lines and Angles Extra Questions which will boost the student confidence and help in solving the exercises and questions from the chapter. The chapter is taken from the new NCERT Mathematics textbook, Ganita Prakash.
These Revision Notes for Class 6 Maths will develop you understanding of the chapter and help in gaining good marks in the examinations. We have also provided Chapter 2 Lines and Angles NCERT Solutions which will help you in completing your homework on time. These NCERT Solutions will help an individual to increase concentration and you can solve questions of supplementary books easily. Students can also check Revision Notes for Lines and Angles Class 6 Maths to prepare for their examination completely.
Important Questions for Chapter 2 Lines and Angles Class 6 Maths
Draw and Label
Question 1. Draw a right angle and label it as ∠ABC.
Answer

Question 2. Draw an acute angle and label it as ∠XYZ.
Answer
Question 3. Draw a straight angle and label it as ∠PQR.
Answer
Fill in the Blanks
Question 1. A line segment has _____ endpoints.
Answer
A line segment has two endpoints.
Question 2. An angle of exactly 90° is called a _____ angle.
Answer
An angle of exactly 90° is called a right angle.
Question 3. A ray starts at one point and extends _____ in one direction.
Answer
A ray starts at one point and extends infinitely in one direction.
Question 4. A protractor is used to _____ angles in degrees.
Answer
A protractor is used to measure angles in degrees.
Question 5. An angle that is greater than 90° but less than 180° is called an _____ angle.
Answer
An angle that is greater than 90° but less than 180° is called an obtuse angle.
True or False
Question 1. A line segment has no endpoints.
Answer
False
Question 2. A straight angle is exactly 180°.
Answer
True
Question 3. An angle bisector divides an angle into three equal parts.
Answer
False
Question 4. A reflex angle is greater than 180° but less than 360°.
Answer
True
Question 5. A ray has two endpoints.
Answer
False
Identify the Image

Question 1. Identify which of the following is a ray.
Answer
C
Question 2. Identify which of the following is a line segment.
Answer
B
Question 3. Identify which of the following is a line.
Answer
A
Figure it Out
Question 1. Draw angles with the following degree measures:
a. 60°b. 45°
c. 180°
Answer
a.
b.
c.
Question 2. Identify the type of angles given below:
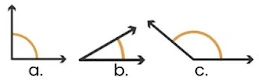
Answer
a. Right Angle
b. Acute Angle
c. Obtuse AngleOne word Answer
Question 1. Measure the angle formed when the hour hand of a clock moves from 3 o’clock to 6 o’clock.
Answer
90°.
The hour hand moves from 3 to 6, which covers 3 hours. Each hour represents 30°, so the total angle covered is 3×30° = 90°.
3 times 30° = 90°.
Question 2. A ray starts at point M and passes through point N. If another point P is on the right of point N on the same line, what is the ray called using these points?
Answer
Ray MP
A ray starts at a point (M in this case) and passes through another point (N), continuing indefinitely through point P, hence it is called Ray MP.
Question 3. If two angles of a triangle are 35° and 65°, find the third angle.
Answer
80°
The sum of angles in a triangle is always 180°. So, 180° − (35°+65°) = 80°.
Question 4. Identify the type of angle (acute, right, obtuse, or straight) that is formed by the hands of a clock at 10:00.
Answer
Obtuse angle
At 10:00, the hands form an angle greater than 90° but less than 180°, making it an obtuse angle.
Question 5. What is the measure of an angle that is three times the measure of a right angle? (1 Mark)
Answer
270°
A right angle is 90°. So, three times the right angle is 3×90° = 270°
Question 6. Two lines intersect and form an angle of 150°. What are the measures of all angles at the intersection?
Answer
The angles are 150°, 30°.
Opposite angles are equal, so two of the angles are 150° and the other two are 30° because 180° − 150° = 30°.
Question 7. Draw a line segment of 5 cm. At its midpoint, draw a perpendicular line segment of 4 cm. What type of angle is formed between the two line segments? (2 Marks)
Answer
Right angle (90°)
A perpendicular line forms a right angle with the original line segment.

Question 8. Can two obtuse angles be supplementary? Explain why or why not.
Answer
No, two obtuse angles cannot be supplementary.
An obtuse angle is greater than 90°. The sum of two obtuse angles would exceed 180°, making them non-supplementary.
Question 9. An angle bisector divides ∠XYZ into two angles of ∠XYB and ∠BYZ. If ∠XYZ=90°, find ∠XYB and ∠BYZ.
Answer
∠XYB = 45°, ∠BYZ = 45°.
The angle bisector divides ∠XYZ equally into two angles, so each is 90°/2 = 45°.
Word Problems
Question 1. Construct an angle of 75° using a protractor and label it ∠ABC. Describe the steps taken.
Answer
Step 1: Draw a ray AB. With A as the center and any radius, draw an arc that cuts AB at C.
Step 2: With C as the center and the same radius, we draw an arc cutting the first arc at D, and with D as the center and the same radius, draw an arc that cuts the first arc at E
Step 3: With E and D as centers and with the same radius, we draw two arcs cutting each other at F. Join AF. The line AF makes 90° with the line segment AB.
Step 4: Mark the point where the line AF touches the semi-circular arc as G. Now with G and D as centers, draw two arcs again cutting each other at H. Now we join AH. ∠HAB= 75°.
The construction of an angle of 75° is shown below:
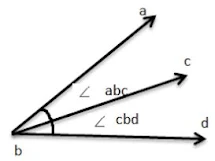
Question 2. If ∠ABC and ∠CBD are adjacent angles where ∠ABC = 25° and ∠CBD= 65°, find ∠ABD.
Answer
∠ABD = 90°.
Adjacent angles are added together to find the larger angle: 25° + 65° = 90°.
Question 3. A triangle has angles in the ratio 1:2:3. Find the measures of all three angles.
Answer
30°, 60°, 90°
Let the angles be x, 2x, and 3x. The sum of the angles in a triangle is 180°. So, x+2x+3x = 180°.
Solving, 6x = 180°, giving x = 30°. Thus, the angles are 30°, 60°, and 90°.
Question 4. Given two parallel lines cut by a transversal with one pair of corresponding angles as 110°, find all other angles formed by the transversal.

Answer
In the given figure, lines m and n are parallel, and line t is a transversal. The question specifies that one pair of corresponding angles is 110°.
Given the figure: Angle 2 corresponds with angle 6, assuming angle 2 is the 110° angle as per the original question.
Angle 2 and Angle 6 are 110° each because they are corresponding angles, and corresponding angles are equal when a transversal cuts parallel lines.
Angle 1 is also110° because angles 1 and 2 are vertically opposite angles, and vertically opposite angles are always equal.
Angle 3, Angle 4, and Angle 5 are 70° each:
Angle 3 is 70° because angles 2 and 3 form a linear pair (they add up to180°).
Angle 5 is 70° as it is corresponding to angle 3 (corresponding angles are equal with parallel lines and a transversal).
Angle 4 is 70° because it is vertically opposite angle 5.
Thus, you have:
- Angle 1 = 110°
- Angle 2 = 110°
- Angle 3 = 70°
- Angle 4 = 70°
- Angle 5 = 70°
- Angle 6 = 110°